A hot Jupiter that can be seen by amateurs

Are monster telescopes in world class facilities and gigantic super-expensive detectors and instruments always needed to do some real science on a planetary system outside of our solar system? After all, I showed in this article that in order to pick out the really faint signals from an exoplanet that would otherwise be lost in the glare of its parent star, professional grade equipment would be needed, of such a scale to dwarf a person standing next to it.

What if that were not the case? What if tuning up an average Meade or Celestron with some fairly mid-grade off the shelf detectors in your own back yard was enough to pick up the signal from a real planet outside our solar system? What if this is all possible from a city location, where dark skies aren't a realistic prospect?

Well, this is precisely what a small team of astronomers did, from the suburbs of London, England, over a decade ago, when small telescopes and detectors weren't as good as they are now.

Let's take a look together at the system they chose to study.

The two stars in the image above are called 'HD 80606' and 'HD 80607' because they were assigned these IDs by a team working at Harvard College Observatory in the early 20th century, funded by the widow of Henry Draper, a pioneering late 19th Century astronomer, and among the first to attempt the classification of the stars.

Both of them golden yellow, a little more orange than our Sun would appear to be, they are reckoned to be separated by a distance of about one thousand two hundred times the Earth - Sun distance. We can be pretty sure of this as they are both close enough for their parallax to be measured, giving a distance of about two hundred and seventeen light years, or roughly sixty six times further away than a star would need to be to have a parallax angle of one arc second.

These numbers might appear to be small, and indeed they place this system in our cosmic back yard - the same 'neighbourhood' of the galaxy (even the same street!), but the distances are still huge. It takes light only one second to travel three hundred million meters. That's three hundred thousand kilometers, or about one hundred and eighty six thousand miles, every second. But that light has to travel that two hundred and seventeen years to reach us.

A close study of these stars' light intensity per wave-length (as seen through a spectroscope), combined with well developed mathematical models of how stars work, has shown us that each star is around about, maybe slightly less than, the mass of our own sun. At their separation, they would then take very approximately thirty thousand years to orbit each other (though no-one has measured this!).

One of these stars - HD 80606 - is orbited by a planet. First discovered as a part of a 1999 survey of nearby stars like our Sun, the planet was detected initially using the giant ten meter Keck 1 telescope by noticing that the star's radial velocity changed over time. A 'radial velocity' is the motion of the star toward or away from the observer, along the radius of a circle that follows the sky, in the direction of the star. Changes in this radial velocity are relatively quick and easy to measure, with a very large telescope.

Given the designation 'b' to distinguish it from its parent star 'a', follow-up observations soon revealed from the way the star's radial velocity behaved over time that the planet was in a highly eccentric orbit. Let's take a moment to discover together what this means for the planet.

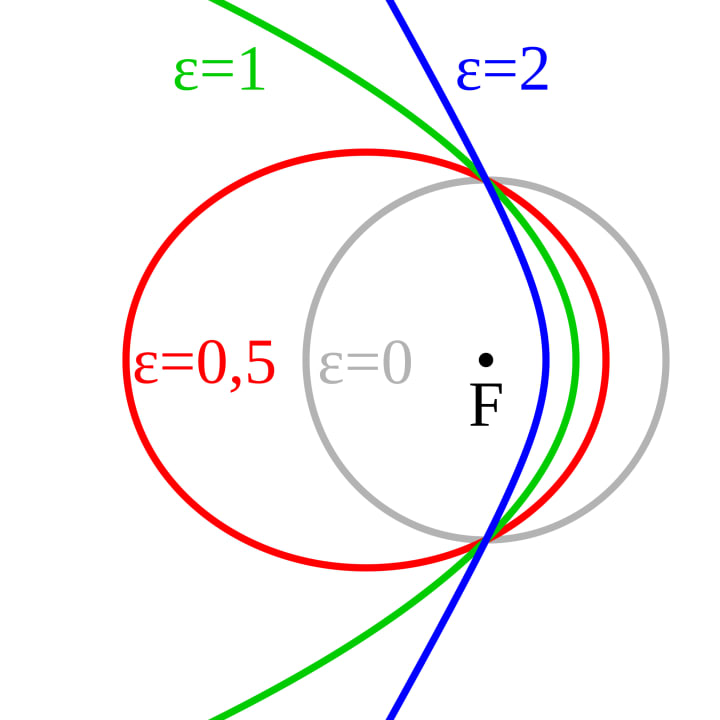
A perfect circle (grey, above) has an eccentricity of zero. If we 'squash' the circle at the top and bottom then it will no longer be a circle. An ellipse is a special kind of squashed circle. Imagine tying two bits of string to two pins stuck into a piece of card along a horizontal line. Now attach both bits of string to a pencil, and draw round both pins as far as possible to form a continuous line. This is an ellipse, and each of the pins is called a focus.

A simple way of thinking about the eccentricity of an ellipse is as the distance from the center of the ellipse to one focus, divided by the distance from the center of the ellipse to the edge. The red line shows an ellipse for which this ratio is one divided by two, or a half. It can be seen immediately that the line of the ellipse is close to the focus 'F' above at one end of the ellipse, and quite far away at the other end. (Technically, the shape for which the eccentricity is exactly one is called a 'parabola' as shown by the green line; for an eccentricity of greater than one it is called a 'hyperbola' as shown by the blue line, but these two shapes can't be drawn in this way).

An exoplanet orbiting a star in an elliptical orbit is a special application of the above - only one of the focus points of the ellipse is occupied - by the star - and the other one is empty. So we can imagine HD 80606 at point 'F', and planet 'b' following the line of the ellipse all the way around as it orbits its parent star.

One of the really amazing things about planet 'b' was that when it was first discovered it was observed to have an eccentricity of about 0.93 - at the time the most eccentric exoplanet ever discovered. This means that the ellipse the planet follows as it orbits its star is really squashed - and the minimum distance of the planet from the star is really very small indeed compared to the opposite side of its orbit. So close to the star, in fact, that it almost 'grazes' the surface! So as the planet coasts past the star at one end of its orbit, it must get really hot. It doesn't do so for long, though, because of the way in which planetary orbits work.

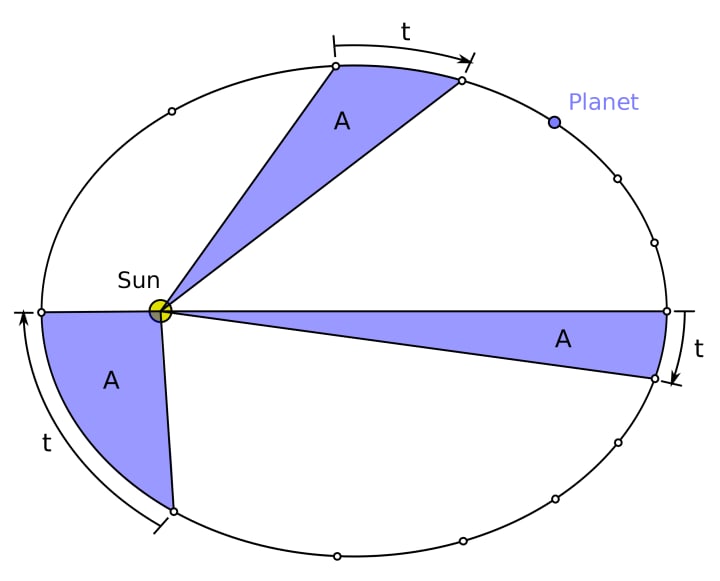
In the diagram above, we're replacing the 'Sun' with the parent star of our exoplanet, HD 80606. We've already covered Kepler's first law above (planets move in ellipses). Now, the the diagram above shows per Kepler's second law that a line connecting the planet with the star sweeps out equal areas in equal time. What does this mean? Well, the orbital period of HD 60606 'b' has been measured as approximately one hundred and eleven days, so let's say that each marked point on the ellipse above represents a ten day interval. Per Kepler's second law, the area 'A' remains constant throughout the orbit of the planet. But the distance moved by the planet in the ten day interval when it is closest to the star is much longer than when it is further away. So this means that the planet 'b' is moving much more quickly when it is closest to the star - it does not hang around in the danger zone for very long.

This illustration shows what can be expected for a planet of mild eccentricity. But HD 80606 'b' has a really extreme eccentricity of 0.93, which means while it approaches its parent star and really roasts, it zips past and soon cools down afterwards. Let's see what this would mean for an observer at the top of the cloud level of the planet as it zooms around the star.

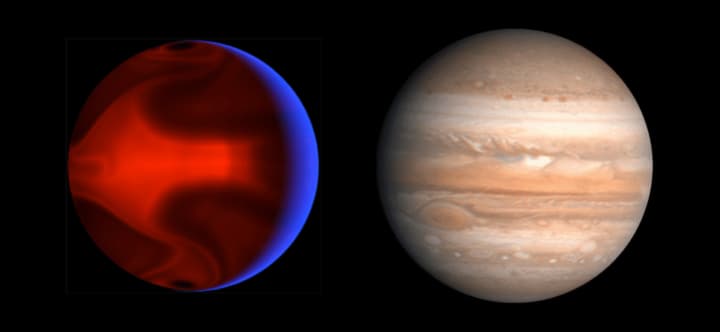
They would see the planet's star swell in size to thirty times it apparent diameter on the other side of the orbit. In just six hours, as the planet approaches its closest point to the star, the temperature at the top of the clouds ascends from around five hundred Celsius (already toasty!) to an astonishing one thousand two hundred Celsius! Such an extreme temperature contrast drives immensely powerful storm winds of eighteen thousand kilometers per hour, many times the speed of sound. Any observer on the night side of the planet would not be safe either: these winds, when coupled with the Coriolis effect from the rotating planet, generate fearsome hurricanes that only die away once the planet recedes and starts to cool.

The planet, on closest approach, zooms past no more than three percent of the Earth - Sun distance. Thankfully, the horrendously challenging environment experienced in the atmosphere doesn't last long, as at the time when the planet is closest to the star, it is moving very quickly.

Follow-up observations of HD 80606 b after initial discovery revealed some more remarkable information.

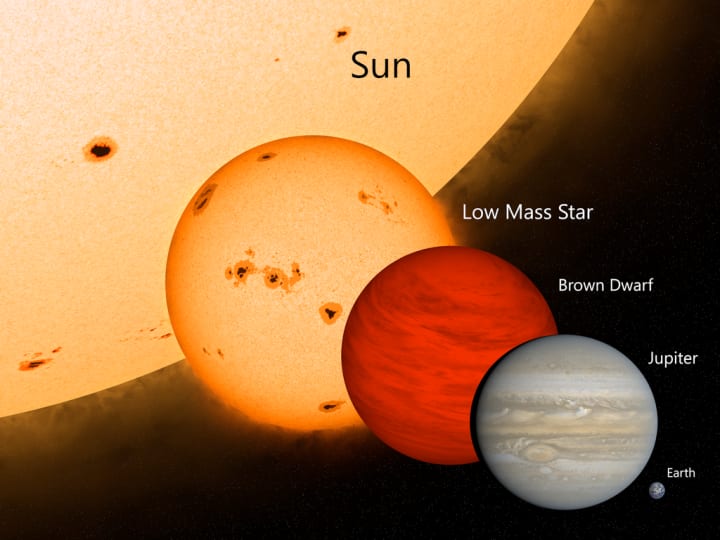
Planet masses are usually expressed as multiples of Jupiter's mass. Jupiter doesn't really emit any light of its own: it reflects light from the Sun. As larger planets accumulate more mass, the central density - and temperature - of the planet increases. Beyond a certain point - thirteen Jupiter masses - the 'planet' starts to glow. Not due to any self-sustaining nuclear reactions, but more due to gradual gravitational squeezing of the core which makes it very hot. Beyond eighty Jupiter masses, nuclear fusion can start in the core, and what was a planet becomes a low-mass star.

The exoplanet HD 80606 b is definitely a planet, of about four times the mass of Jupiter. It would need to be three times more massive than that to be a brown dwarf. One surprising thing about this though is that the diameter of the planet hardly increases at all as more mass is added. Indeed, observations have shown (see below) that the diameter of the planet is very approximately the same as Jupiter's; maybe a little larger.

Also remarkable is the inclination of the planet's orbit relative to the plane of rotation of the star. A bit like the orbit of Pluto, HD 80606 b rotates around its star at an angle. It is possible that the combination of the planet's high eccentricity and high inclination could be explained by an interaction between the other star in the binary, HD 80607, and the planet: every so often, the other star 'pulls' the planet slightly further out from what was initially a nice circular orbit a safe distance from its star, via a effect known as the Kozai mechanism. Gradually, over time, the point of closest approach is driven closer and closer to the star, until today when it is periodically fried while zipping past. No-one really knows what the underlying reason is though: more research is needed.

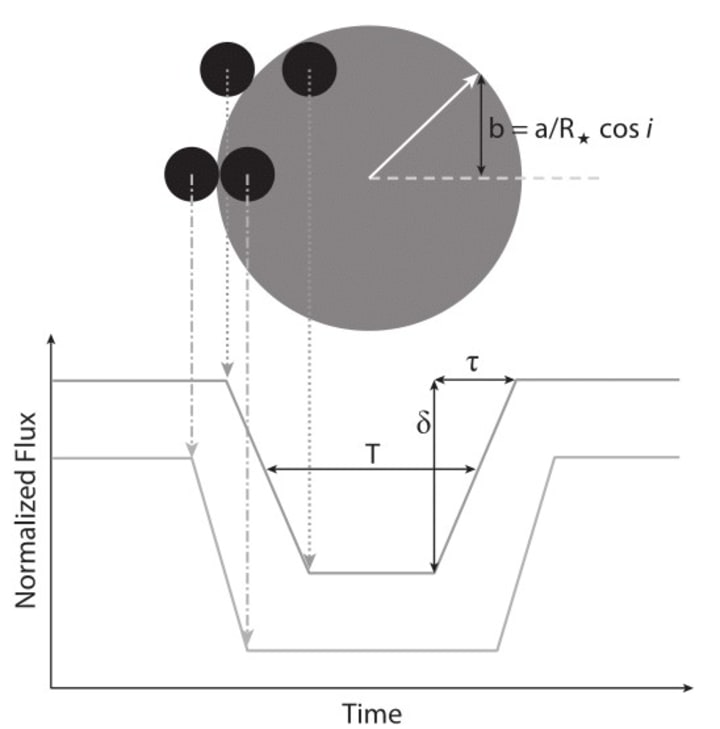
There's one more incredibly neat thing about this system - it transits in front of its parent star, partially eclipsing some of its light. It is this characteristic that makes it so accessible to amateur observers, helped of course by the parent star being 'relatively close' and of ninth magnitude in the sky.

As the planet passes in front of the star, some fraction of the light of the star is temporarily blocked. The changes in the brightness of the star over time is called the light curve. As the planet is relatively small compared to the star, the loss of light isn't that great (no more than one percent or so) but that change is more than enough to be picked up by today's modern sensitive detectors on small-ish amateur telescopes.

There's a lot that can be learned from the transit of the exoplanet in front of HD 80606. The slope or gradient of the ingress (left hand side) and egress (right hand side) of the planet in front of the star measures the size of the planet relative to the star. If we know the size of the star (from its spectral type) then we know the size of the planet. That enables us to work out the density, showing whether this is a rocky planet, or a gas giant. Measuring the length of time the transit lasts, as well as precisely when it starts and ends, enables us to plot the orbit more precisely, and to constrain the inclination of the system. As well as of course to measure the mass of the planet more precisely.

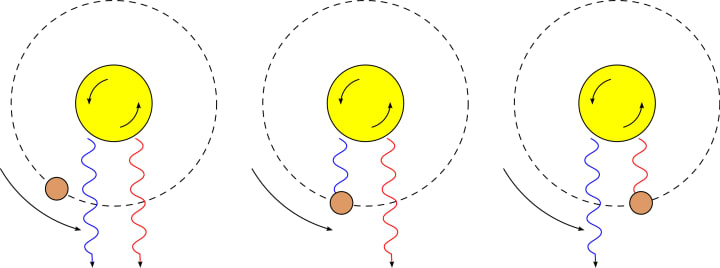
A fundamental question needing to be addressed first is how do we know the temporary dimming of the star's light is due to a planet passing in front of it. One independent way in which we can confirm this is what is happening is by measuring the way in which the precise wavelength of spectral lines change as the planet transits the star. Most stars rotate, some more than others. As the planet begins its transit, it blocks some of the light from the limb of the star on the left, coming towards us. As that light would appear to be blue-shifted (via the Doppler effect) then the net result is we see a red-shift in the spectral lines. The same thing happens in reverse as the planet completes its transit. Measurements of the light from HD 80606 during a transit show not only this effect happening, but also that the planet is orbiting prograde (in the same direction) relative to the star's rotation.

The small team of observers were able to record this very small change in the light of the star, from a location literally right next to a six lane highway in a busy urban location with Bortle 7 skies. Skies in which the light pollution reaches all the way to the zenith, clouds (when present!) are brilliantly lit, and it is almost possible to read a newspaper by ambient skylight.

They were able to observe most of the transit that occurred on the night of 14 February 2009, including the egress, using two telescopes that they cross-correlated with each other. The first one was like the picture shown above, but somewhat larger, at a fourteen inch aperture.

The other, that was used more as a backup, was more or less like the Meade LX200 shown above.

Images of the field in which HD 80606 and HD 80607 sit were taken repeatedly over the night - ten second exposures every twenty seconds on the larger telescope, and thirty seconds with an additional five second read time on the smaller telescope.

The team used standard image reduction techniques using software similar to that which an amateur could download and install. This included treatment with bias frames, darks and flat fields to ensure their images were as free from systematic artefacts and read-out noise as possible.

They then used similar software to 'draw' a circle around HD 80606, as tightly as possible, to exclude any contamination from its nearby binary companion, and carefully measured the light within this circle. The number they arrived at was plotted on a graph of 'brightness' versus time to achieve the light curve of the star as the exoplanet completed its transit and egress.

For consistency checking - to make sure that the small variations they were seeing weren't due to some other effect, like thin wispy cloud, they also recorded the brightness of several other stars in the frame, including that from the companion HD 80607 that we know doesn't change intrinsically.

From these observations, the team were able to measure the egress duration, the total duration of the transit, the ratio of diameters of the exoplanet and the star, the inclination of the orbit of the planet relative to the line of sight, and therefore the planetary radius, to within one percent.

Quite a remarkable achievement, using amateur equipment in a city site next to a highway. And a truly remarkable exoplanetary system.

Let's face it, if they can do it, so can you.